
Image: STUPID: Reuters Daydreams ‘Nobel Peace Prize’ for Greta Thunberg (Don’t get surprised, we all know who’s on the Noble Institute’s Peace Prize committee in Oslo, most of them are left leaning activists, previously corrupt politicians.)
Related, but not supported by science: World Leaders Fear End of Times: Tell U.N. to Act on Coronavirus and Climate or Face Doom
No Tricks Zone’s Erich Schaffer is a bit optimistic, if the (Man Made) Global Warming (what they now call “Climate Change” due to lack of warming) BS was about science the nonsense would have ended in 1909 – and stayed dead ..
By Erich Schaffer – No Tricks Zone
Magic is all about illusion, and so is “climate science”. Nothing about the GHE is as it seems and so far the “critical” side has failed to see through it. No longer!
Examining the key question of surface emissivity, usually downplayed as a non-issue, reveals highly significant insights relativizing the common narrative and revealing another profound flaw in the theory. Eventually this will lead to a totally disruptive Eureka moment.
Did you know that even the moon has a GHE? If we adapt our “GHE formula” to the circumstances on the moon, we can easily calculate the maximum temperature it should yield at the equator (with the sun at the zenith). All we need to do is to allow for the specific albedo (~0.12) and the amount of solar radiation, which is about 1368W/m2.
(1368 * ((1-0.12) / 1) / 5.67e-8) ^0.25 = 381.7°K
Since the albedo is a bit uncertain (in the 0.11 to 0.13 range), let us just say 382°K straight. So the moon, at the equator, should theoretically yield a temperature of up to 382°K. The observed (average) maximum temperature however is about 394°K [1][2][3][4], 12°K hotter than it should be. The same formula that tells us Earth had a GHE of 33°K, suggests 12°K of GHE on the moon. Houston, there is a problem! By the way, if the albedo was 0.3, like that of Earth, the formula would give us 360.5°K in this case, with a GHE of 33.5°K. Sounds strangely familiar, right?
Of course we know there is NO GHE on the moon, since it does not hold an atmosphere. Instead there must be something wrong with our theoretical approach, the formula itself. And if the very formula that determines the GHE of Earth is wrong, as just demonstrated, it might be about time to ring the alarm bells.
What went wrong?
There are two ways to get to the correct result with regard to the moon. First we could simply ignore the albedo, or set it to zero respectively, and then the formula gives the correct result of 394°K. This is pretty odd, since we know the albedo is real and ignoring it is wrong, and yet it seems to work. That is why the second approach makes way more sense. Next to including an albedo of 0.12, giving us an absorptivity of 0.88, we also assume an emissivity of 0.88, so that the two cancel each other out. Then again the result is 394°°K and everything is fine. In fact, an emissivity of 0.88 is the only value consistent with an albedo of 0.12 and an observed temperature of 394°K.
The mistake we initially made is easy to identify. We started with assuming an emissivity of 1, while in reality it is far lower. Such a mistake will always give you a calculative temperature which is too low, and thus an erroneous “GHE”. Bearing that potentially fatal mistake in mind, how do leading climate experts around the globe account for it? They say emissivity is 1, or so close to 1 that it is negligible, and certainly there was no reason to question it any further.
This unawareness carries well into the “critical” arena, where the question would usually be resolved by looking up text books. It turns out, this is totally insufficient since a) text books are mostly wrong and b) it does not help understanding the nature of the problem. It is pretty odd, given that what I am going to reveal is really climate science 101.
If you don’t know that, you know nothing! And it seems no one knows.
So what is the surface emissivity?
It should be easy to answer since NASA is operating some research projects on the subject, with satellites “measuring” surface emissivity. Measuring may be a bit too much, since the actual data need interpretation in the context of models and so on. Anyhow, those measurements show a quite significant deviation from 1, especially with arid regions.[5]:

Now we would only need to calculate a weighted average of these data and we would finally have a definite answer on what surface emissivity of Earth is, right? WRONG!
Regrettably it is far more complicated, and, spoiler alert, ironically much simpler eventually in the end (we’ll get to that shortly).
Inadequate global surface emissivity data
There are two huge issues with these data. First, quite obviously, water has been completely exempt and since it covers 71% of the planet, we can not ignore it. Second, the sensors usually top out at 15 µm (or less) and that leaves around 50% of the emissions spectrum at 288°K unchecked, as the chart below shows (black being the uncovered range).

The satellite data thus only cover 50% of 29%, or about 15% of global surface emissivity, giving zero information on the remaining 85%. This is totally insufficient, like predicting the US presidential election by only polling California. However, the problems we encounter ironically also lead the way to a solution.
A sunset over water is a beautiful sight. You see the sun over the horizon, and a strong reflection of the sun in the water. It would never look anything like it if the sun was higher up in the sky. Of course there is a reason for it, a law of physics, and that is exactly what we are after.
Fresnel equations allow us to determine precisely how much light is getting reflected on the surface of water, depending on the angle of incidence. I will not try to explore the formulas themselves, as they look quite complicated. Thank God they are not so hard to apply. All you need to know is the refractive index of water with regard to visible light (N = 1.33)[6][7], that of air (N=1) and then you get two results for s- and p- polarized light. Then you just take the average of those two curves and you get the final result.
A chart tells us more than a thousand words:

With the sun at the zenith, or anything near to it, water reflects only 2% of sunlight. But if the sun is close to the horizon, shining onto the water at a flat angle, most of sun light gets reflected, which is one of the reasons why sun sets look so beautiful.
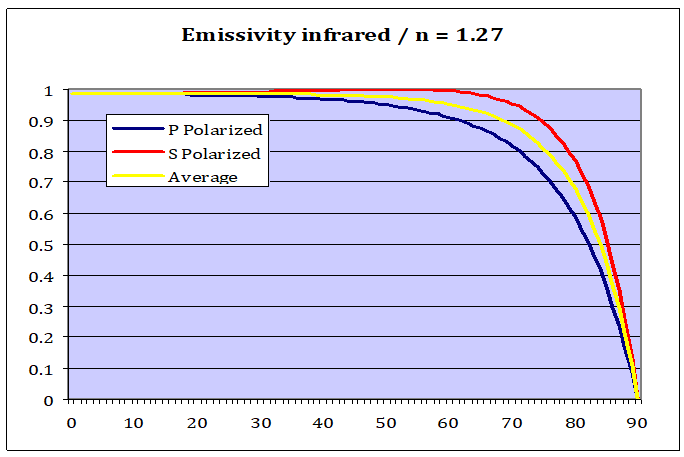
Physically speaking sunlight is nothing but electromagnet radiation, just like LWIR, and thus the same physics apply. We only have to adapt the formula slightly to show how well water emits LWIR. First we need to know the appropriate refractive index in the LW range (N=1.27)[9] and then we just invert the result, since we do not search for radiation reflected, but rather what is being emitted.

As we can see water is an excellent vertical emitter, with an emissivity of 0.986. This number so close to 1 that you could say the difference was indeed negligible. But that is only part of the truth. With flatter angles the picture changes dramatically and finally turns to 0 emissivity towards the horizon.
Satellites do not measure water emissivity
At this point it should become clear why satellites do not measure water emissivity. To minimize atmospheric disturbance, satellites need to look straight down onto the surface, which in this case is kind of pointless. The more interesting data could only be gathered by looking “sideways”, which satellites are very bad in. Even then, water is a very homogeneous surface type, which renders global observation obsolete, while on the other side we a have a solid theoretic approach. As far as empirical testing is concerned, 10 years ago “scienceofdoom” made a nice summary of research on the subject.[10]
If you google “emissivity of water” you will get all kinds of results. Given the complexity of the subject (including the political aspect) it is quite understandable. Regrettably this means you will have to figure it out yourself, which is mainly a mathematical challenge. The Fresnel equation tells us how water emits at a certain angle, but to get the hemispheric emissivity (we live in a 3 dimensional world) you will need to weight every gradient accordingly and also include Lambert’s cosine law. If I do all that, the result is 0.944.
Is this the final answer? I am not so certain! Anyone good in physics, or mathematics is invited to assist. One of the causes of doubt is, that German wikipedia [11], with reference to a noble German text book[12], has it at 0.91. Maybe I made a mistake after all. Then, just recently, I stumbled over an article stating “the emissivity of far-IR by the oceans is only about 89% of the 100% “efficiency” of emission/absorption of a true blackbody”[13], thereby suggesting a relatively larger refractive index in the far-IR (15-100µm). So I started again and with the help from a very useful site[14] calculated the average refractive index for the whole LWIR spectrum, which turned out to be N = 1.33, exactly the same as with SW radiation. With N = 1.33 my result for hemispheric emissivity drops to 0.934. By the way, I get 0.9 or 90% for the 15-100µm of far IR range, still relatively consistent with the 89% quoted above.
Kiehl/Trenberth fake science
All this may sound rather complicated and in the end there is still this sour taste of uncertainty. We know for sure however, that surface emissivity, especially with regard to water, is significantly lower than 1. With an emissivity of 0.94 a surface at 288°K emits 367W/m2, not 390. This alone shoves off 23W/m2 from the GHE at least and exposes the infamous “Kiehl, Trenberth diagram”, where they claim 390W/m2 of surface emission (396W/m2 in later editions), as fake science. That’s it!
Did I forget something?
Oh yes, the big revelation. At this point we achieved more than we ever imagined, we just have not realized it yet. If we wind back to our Fresnel charts, there is an opportunity we missed. I did show reflectivity of water with regard to solar radiation and if we invert the chart, we get the result for absorptivity.
In the logical next step, we can directly compare absorptivity with emissivity and, as we can see, they are almost identical. That is if we assume N = 1.27 for LWIR, which is in line with experimental observations which exclude far-IR. As named before, including far-IR would move N likely up to 1.33, with absorptivity and emissivity then being perfectly identical.

What is the temperature of a body when absorptivity and emissivity are equal at given solar radiation?
(342 * (x / x) / 5.67e-8) ^0.25 = 278.7°K
GHE only 10°K
In other words, if there was no atmosphere, just the surface as it is (excluding any dynamic changes, ceteris paribus), the surface of Earth would take on the temperature of 278.7K. If we allow for N = 1.27, thus the little asymmetry between the curves, the temperature would drop straight by 0.7°K to 278°K . Without an atmosphere, Earth would be 10°K colder.
Or, alternatively spoken, the GHE is 10°K in size!
It is a revelation you may want to let sink, or protest respectively, but it is true. Just like with the initial example of the moon, the surface of Earth absorptivity and emissivity largely cancel each other out. This is not a law of physics, as Kirchhoff’s law only applies to identical wave lengths. But as much as there is variation in both absorptivity and emissivity with different wave lengths, the general tendency is for both of them to be more or less equal, especially with regard to wide spectra.[15]
Plenty of flaws – huge GHE hoax
The 33°K GHE narrative by comparison is build on a few “imprecisions”, so it seems. There is the surface emissivity = 1 mistake, there is the formal impossibility of counting cloud albedo to the “surface”, while at the same time denying the sheer existence of clouds in terms of emissivity. And then there are also plenty of flaws in the quantification of radiative effects, especially with regard to the CRE, that I have dealt with deeply in the previous article. All these “imprecisions” point in the same direction, certainly not accidentally so, and all together they accumulate to a huge GHE hoax.
It is a hoax as far as the apologetic side of “global warming” is concerned, but for the “critical” side it is an appalling failure. I have absolutely no understanding for how you can deal with the fiddliest questions of the science, polar bear populations, or Greta Thunberg’s pigtails, but totally miss out on the core blunder.
Difference between GHGE and GHE
To be accurate, the GHGE (greenhouse gas effect) needs to be distinguished from the GHE itself. The 278°K are true for the surface alone, and the 10°K GHE is what the atmosphere does as a whole. Of course it is perfectly thinkable there are components within the atmosphere which reduce the temperature of Earth, and then you can attribute more than just 10°K of GHE to GHGs.
If for instance clouds would cool the planet by 5°K, then we would arguably need 15°K of GHGE to get us back up to 288°K in the end. The global warming narrative thus interestingly takes relatively little harm, and can still prevail. The only precondition it needs is a significant net negative CRE. That of course is another story.
Footnotes
[1] https://lunarpedia.org/w/Lunar_Temperature
[2] https://www.nasa.gov/pdf/203096main_TEC%20Splinter-Thermal%20control.pdf
[3] https://www.sciencedirect.com/science/article/pii/S0019103516304869
[4] Actual maximum temperatures can reach over 400K, which is due to some craters serving as a kind of parabolic reflectors
[5] https://www.mdpi.com/2072-4292/10/7/1027/htm
[6] https://pixabay.com/de/photos/hamilton-island-australia-sun-set-585659/
[7] https://en.wikipedia.org/wiki/List_of_refractive_indices
[8] https://refractiveindex.info/?shelf=main&book=H2O&page=Hale
[9] N=1.27 is consistent with measurements on the subject. These measurements yet exclude the far long wave range. A total weighted spectral average according to the data here (https://refractiveindex.info/?shelf=main&book=H2O&page=Hale), would rather elevate N to 1.33, meaning identical Ns for LW and SW radiation.
[10] https://scienceofdoom.com/2010/12/27/emissivity-of-the-ocean/
[11] https://de.wikipedia.org/wiki/Emissionsgrad
[12] H. D. Baehr, K. Stephan: Wärme- und Stoffübertragung. 4. Auflage. Springer-Verlag, Berlin 2004, ISBN 3-540-40130-X (Kap. 5: Wärmestrahlung)
[13] http://hockeyschtick.blogspot.com/2014/11/new-paper-finds-huge-false-physical.html
[14] https://refractiveindex.info/?shelf=main&book=H2O&page=Hale
[15] Ironically NASA fully acknowledges this. NASA does not really measure the surface temperature of the moon, rather they measure radiation emitted with a satellite. To determine the actual temperature, they need to assume a certain emissivity. Essentially they simply reverse the formula I used above. The only way they can get to 394°K is by assuming absorptivity = emissivity. And they need to do this in order to get reasonable scientific results. When it comes to “climate science” however, they forget about science and stick rather to the emissivity = 1 myth.
Ref.: https://notrickszone.com/2020/09/27/plenty-of-physics-flaws-accumulate-into-a-huge-ghe-hoax-the-dark-secret-behind-surface-emissivity/
2020: A Year Of Global Surface Cooling… And Sun Showing Little Activity, Spotless 30 Days

And totally out of touch with reality, protected from any critical scrutiny by the complicit left leaning and corrupted media:
Soros/Gates Funded Progressives Planning ‘Climate lockdown’ – ‘The world may need to resort to lockdowns again – this time to tackle a climate emergency’
The real world just doesn’t exist!


